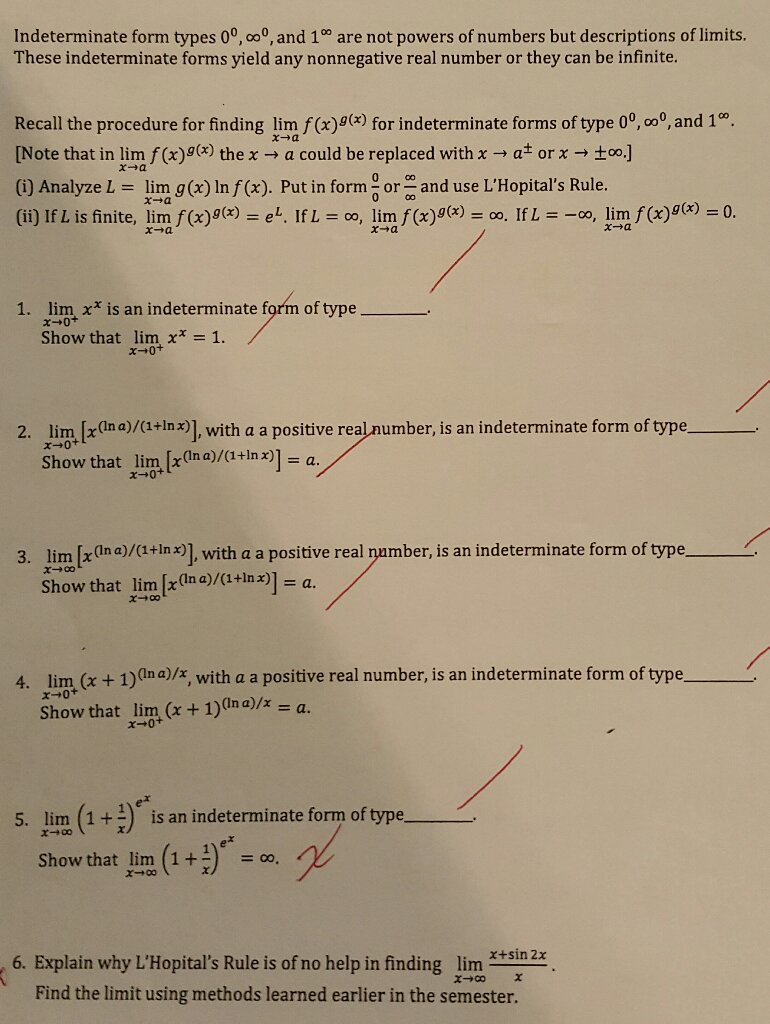0 Infinity Indeterminate Form

0 Infinity Indeterminate Form - An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. If $f(x)$ approaches $0$ from below, then the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. Specifically, if $f(x) \to 0$ and $g(x). The process of finding the.

L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. The process of finding the. If $f(x)$ approaches $0$ from below, then the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. Specifically, if $f(x) \to 0$ and $g(x).

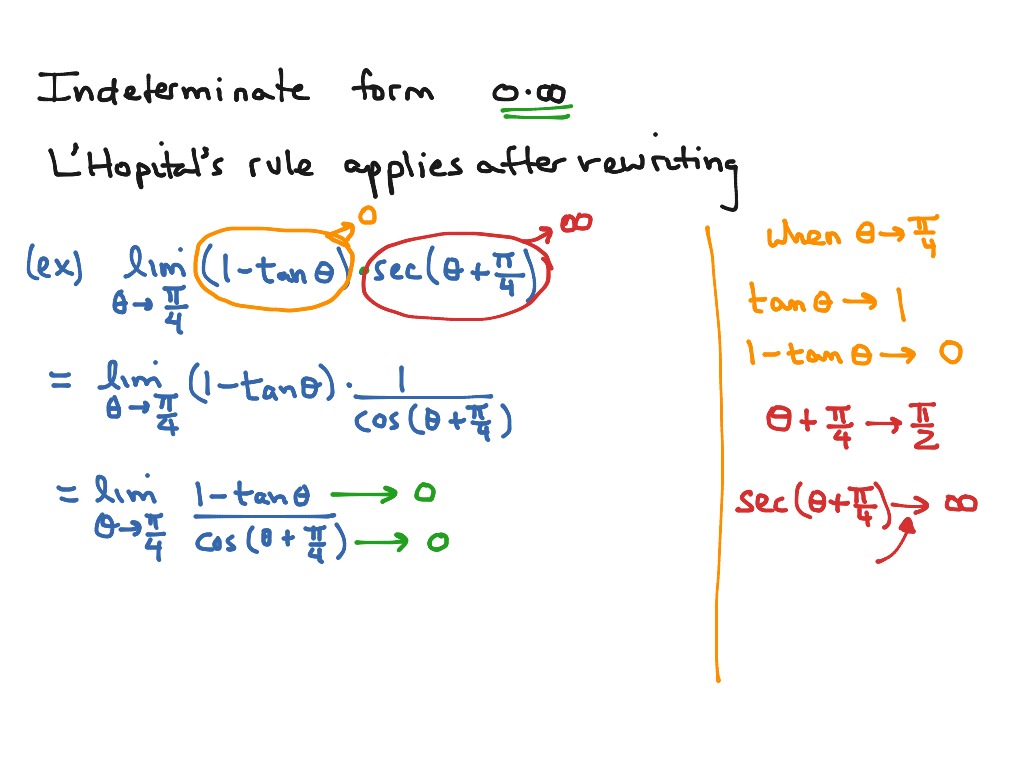
You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. If $f(x)$ approaches $0$ from below, then the. Specifically, if $f(x) \to 0$ and $g(x). If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. The process of finding the. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined.

Indeterminate form types 0^0, infinity^0, and

If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. If $f(x)$ approaches $0$ from below, then the. Specifically, if $f(x) \to 0$ and $g(x). L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's.

6.9 Indeterminate form ZERO times INFINITY YouTube

You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. Specifically, if $f(x) \to 0$ and $g(x). L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. If $f(x)$ approaches.

What Is Infinity Multiplied By 0

An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. L’hospital’s rule.

Indeterminate Form 0 to 0 YouTube

The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. Specifically, if $f(x) \to 0$ and $g(x). If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. If $f(x)$ approaches $0$ from below, then the.

Indeterminate form 0*infinity example Math, Calculus, Limits, 0

Specifically, if $f(x) \to 0$ and $g(x). An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from below, then the.

Indeterminate Form Infinity Infinity YouTube

L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. The process.

Finding Indeterminate Limits L'Hôpital's Rule 0/0, infinity

If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. Specifically, if $f(x) \to 0$ and $g(x). If $f(x)$ approaches $0$ from below, then the. The process of finding the.

Indeterminate form 0 times INFINITY YouTube

You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. If $f(x)$.

Calculus Indeterminate Forms YouTube

Specifically, if $f(x) \to 0$ and $g(x). An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. If $f(x)$ approaches $0$ from below, then the. L’hospital’s rule works great on the two indeterminate forms 0/0 and.

Solved Show that 0 infinity is not an indeterminate form by

You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from below, then the. L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. Specifically, if $f(x) \to 0$ and $g(x). The process of finding the.

The Process Of Finding The.

You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. Specifically, if $f(x) \to 0$ and $g(x). L’hospital’s rule works great on the two indeterminate forms 0/0 and ${{ \pm \,\infty }}/{{ \pm \,\infty }}\;$. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined.