Image In Math Definition
Image In Math Definition - The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos and get hints on. Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes.
The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos and get hints on. Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes.
The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Watch videos and get hints on. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes.
What Is Expression Tree In Data Structure Design Talk
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos and get hints on. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is.
Definition of mathematics YouTube
The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos.
Grouping Symbols in Math Definition & Equations Video & Lesson
Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Watch videos.
Explain Math
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos and get hints on. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is.
Range Math Definition, How to Find & Examples, range photo
The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos.
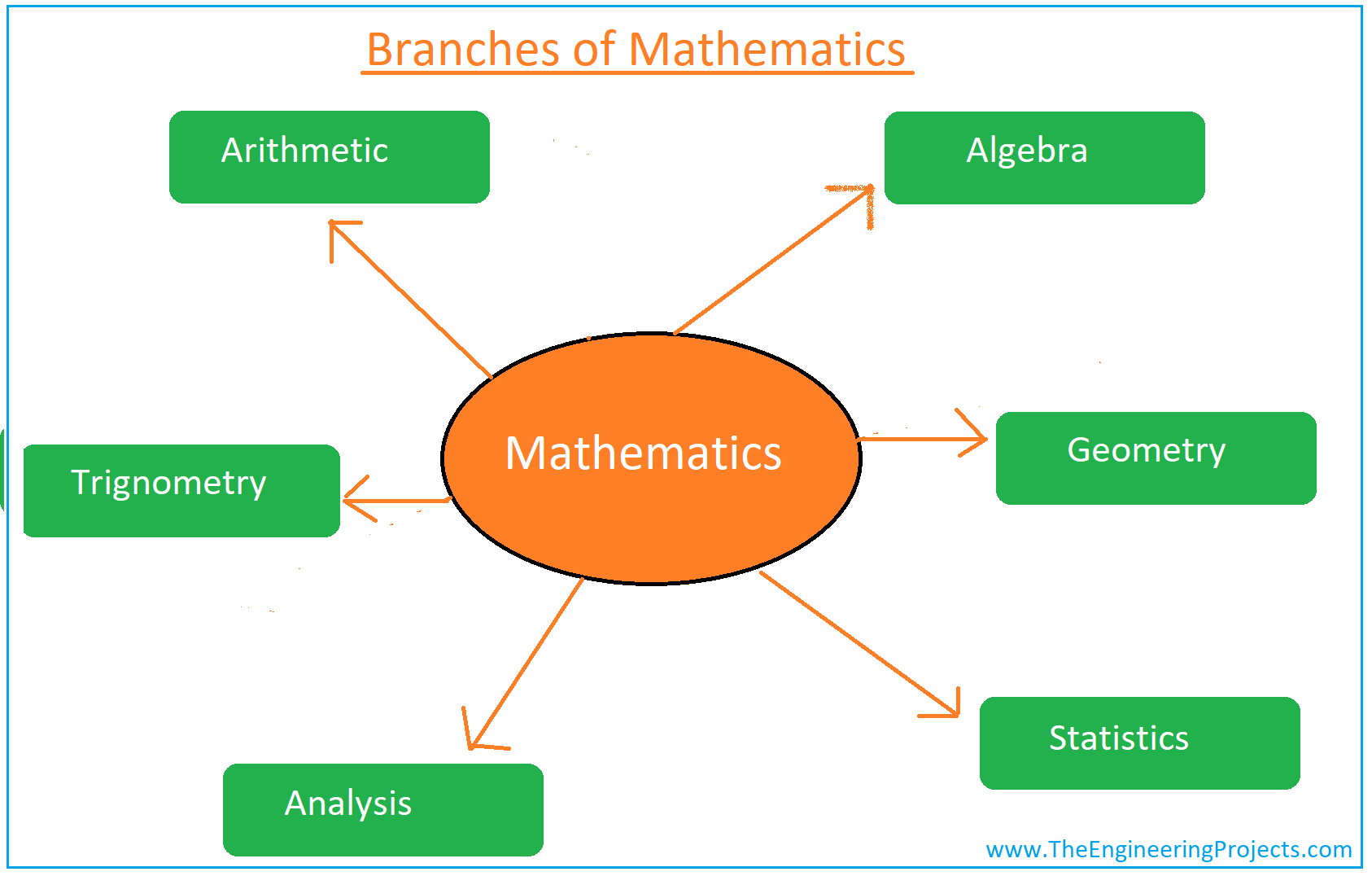
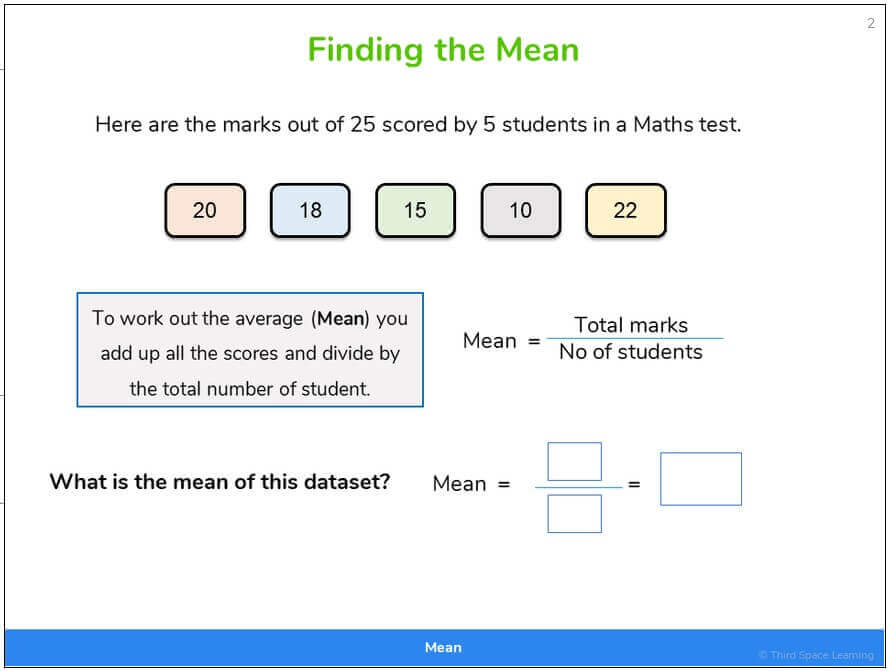
Math Mean Definition
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Watch videos and get hints on. Learn what an image is in math, the new figure you get when you apply a.
Identity Property in Math Definition and Examples
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. Watch videos and get hints on. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is.
Math Mean Definition
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Learn what an image is in math, the new figure you get when you apply a transformation to a figure. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Watch videos.
Math Mean Definition
Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Watch videos and get hints on. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Learn what an image is in math, the new figure you get when you apply a.
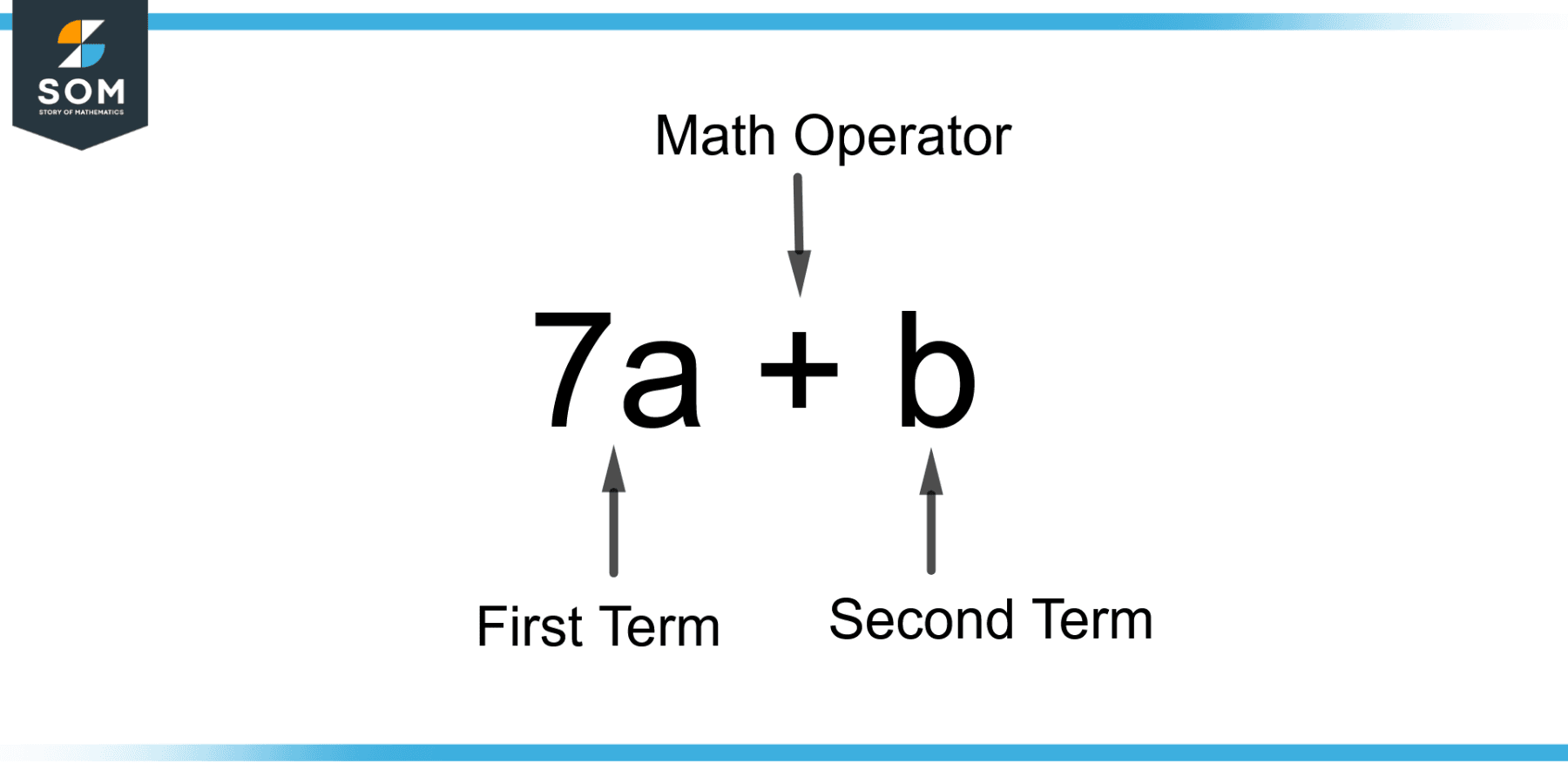
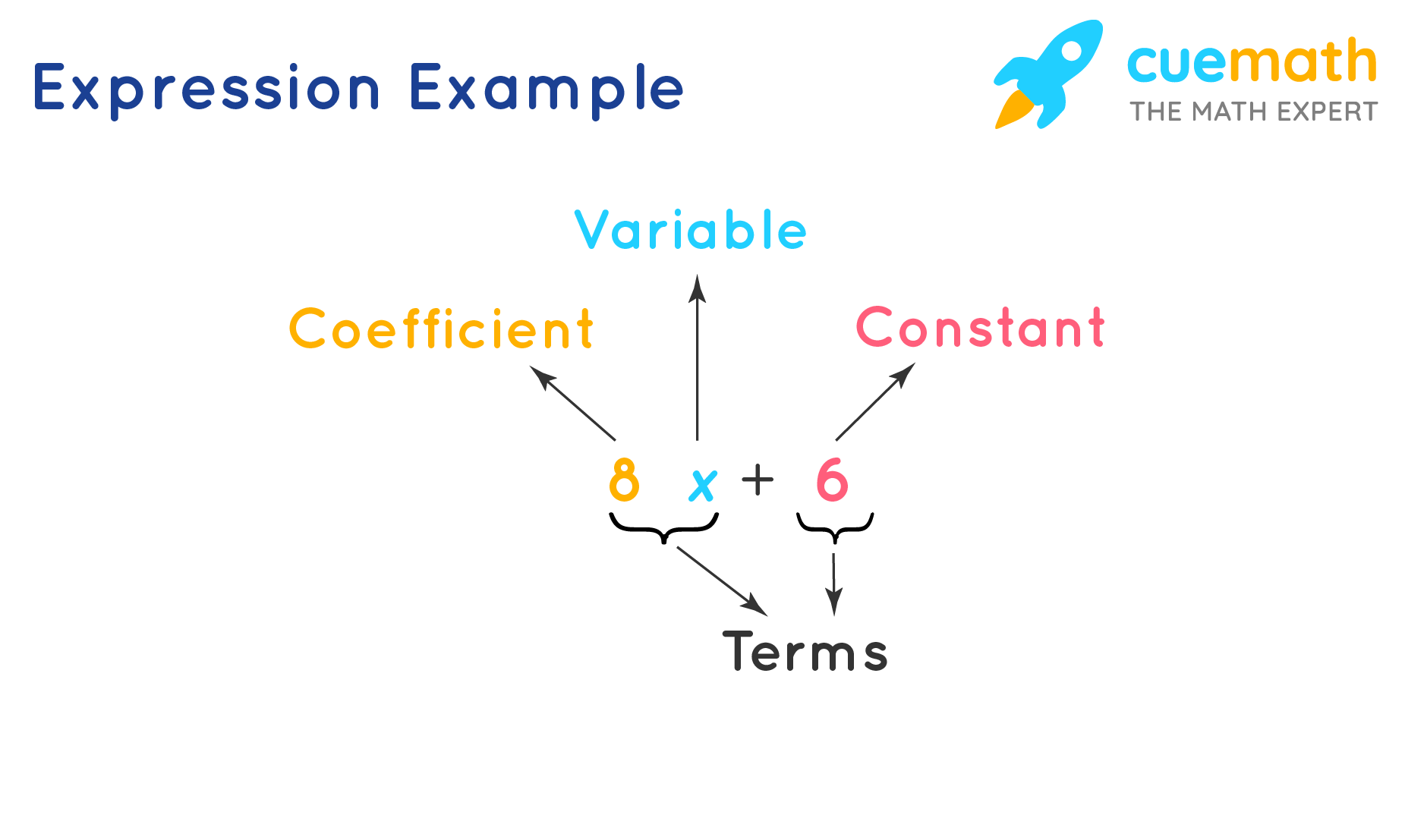
Like Terms Math Definition
Learn what an image is in math, the new figure you get when you apply a transformation to a figure. The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Images are pivotal in computing homology groups as they define which elements contribute to cycles and boundaries within chain complexes. Watch videos.
Images Are Pivotal In Computing Homology Groups As They Define Which Elements Contribute To Cycles And Boundaries Within Chain Complexes.
The image of \(a_{1}\) under \(f\) is \[f\left(a_{1}\right)=\left\{f(a) \mid a \in a_{1}\right\}.\] it is a subset of \(b\). Watch videos and get hints on. Learn what an image is in math, the new figure you get when you apply a transformation to a figure.


:max_bytes(150000):strip_icc()/statistics-ae8f1320de174c279eeeee49c4087917.jpg)