Relations Discrete Math
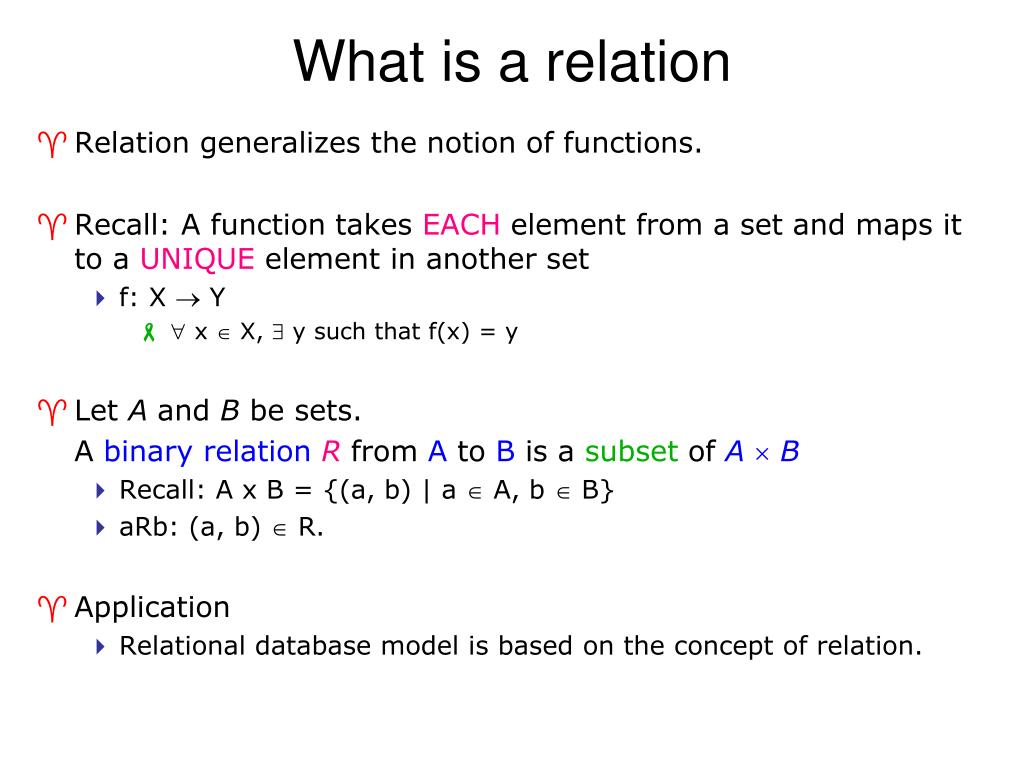
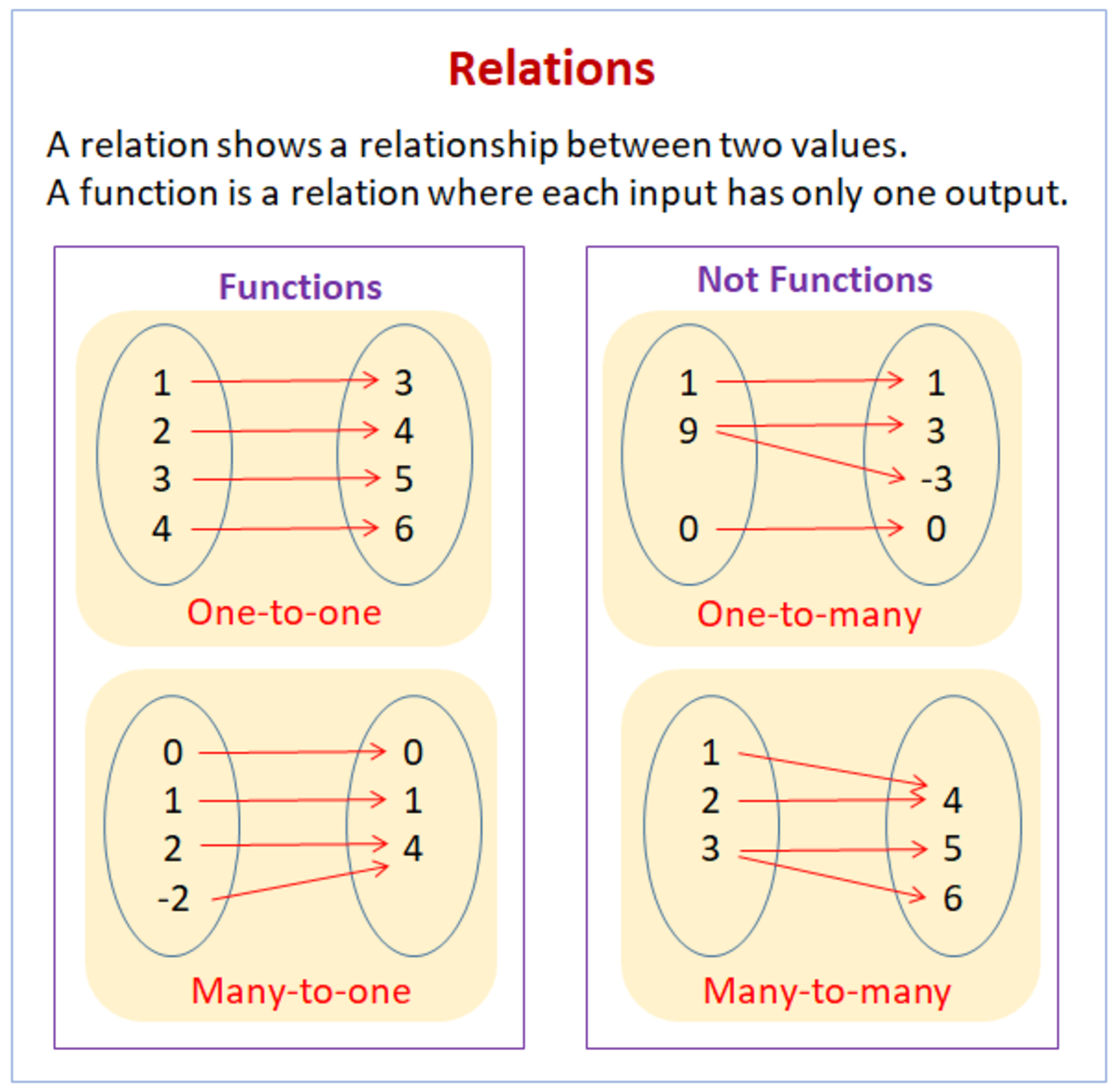
Relations Discrete Math - Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. Given any relation \(r\) on a set \(a\), we are interested in five properties. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\).
Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. Given any relation \(r\) on a set \(a\), we are interested in five properties. Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\).
Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. Given any relation \(r\) on a set \(a\), we are interested in five properties. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\).
Relations Example 1 (Discrete Maths) YouTube
A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\).
PPT Discrete Mathematics Relations PowerPoint Presentation, free
Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\) to itself is called a relation on \(a\). Given any relation \(r\) on a set \(a\), we are interested in five properties. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of.
Discrete Math Relations (Illustrated w/ 15 Examples!)
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\).
Introduction to Relations YouTube
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Given any relation \(r\) on a set \(a\), we are interested in five properties. Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation.
Kinza Javaid on HubPages
Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. Given any relation \(r\) on a set \(a\), we are interested in five properties. Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\).
Discrete Mathematics Introduction to Relations YouTube
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\) to itself is called a relation on \(a\). Given any relation.
Discrete Math Relations (Illustrated w/ 15 Examples!)
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to itself is called a relation.
PPT Discrete Mathematics Relations PowerPoint Presentation, free
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. Hence, a relation.
Discrete Math Relations (Illustrated w/ 15 Examples!)
A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\),. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation.
Discrete Math Intro to Relations YouTube
Given any relation \(r\) on a set \(a\), we are interested in five properties. Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). A relation from a set \(a\) to itself is called a relation on \(a\). A.
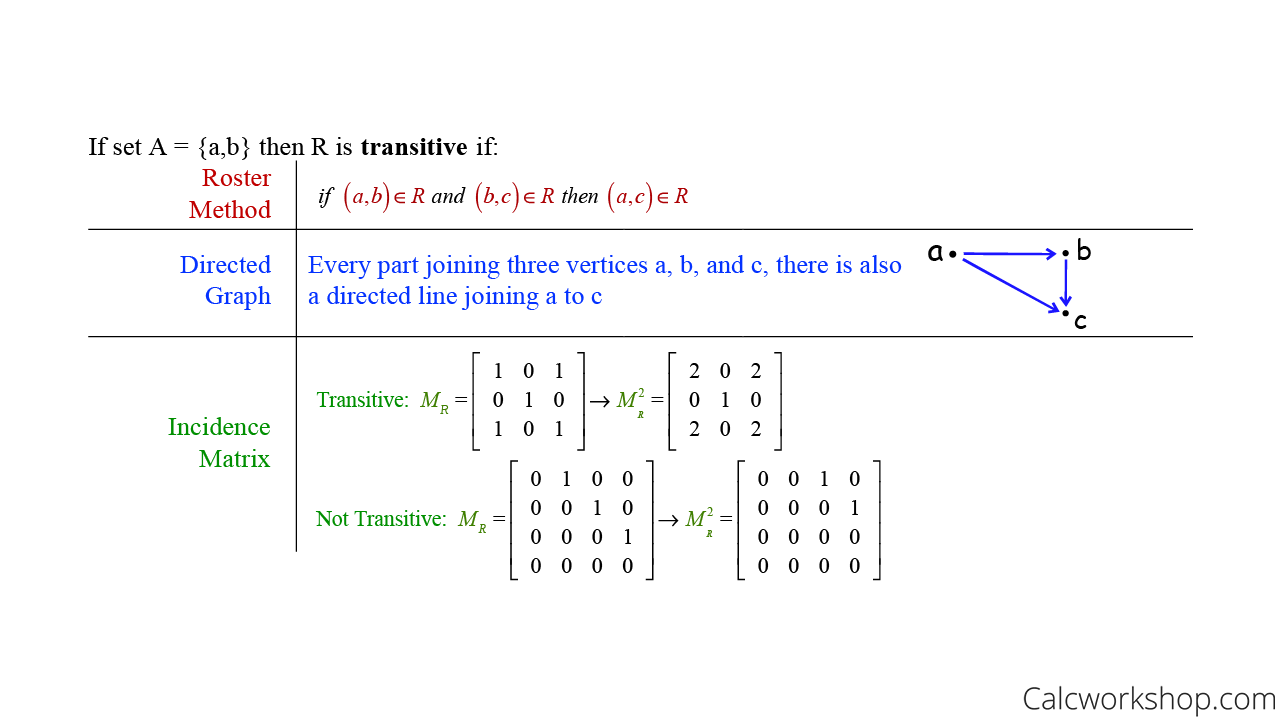
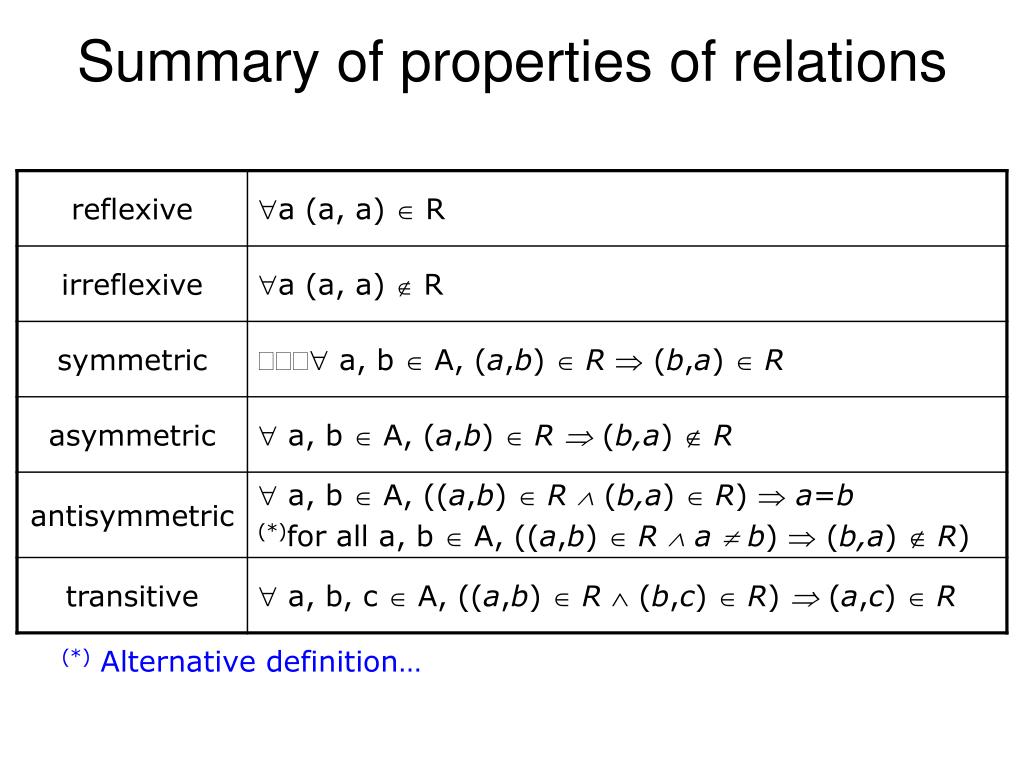
Given Any Relation \(R\) On A Set \(A\), We Are Interested In Five Properties.
A relation from a set \(a\) to itself is called a relation on \(a\). A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\). Hence, a relation \(r\) consists of ordered pairs \((a,b)\), where \(a\in a\) and \(b\in. A relation from a set \(a\) to a set \(b\) is a subset of \(a \times b\).