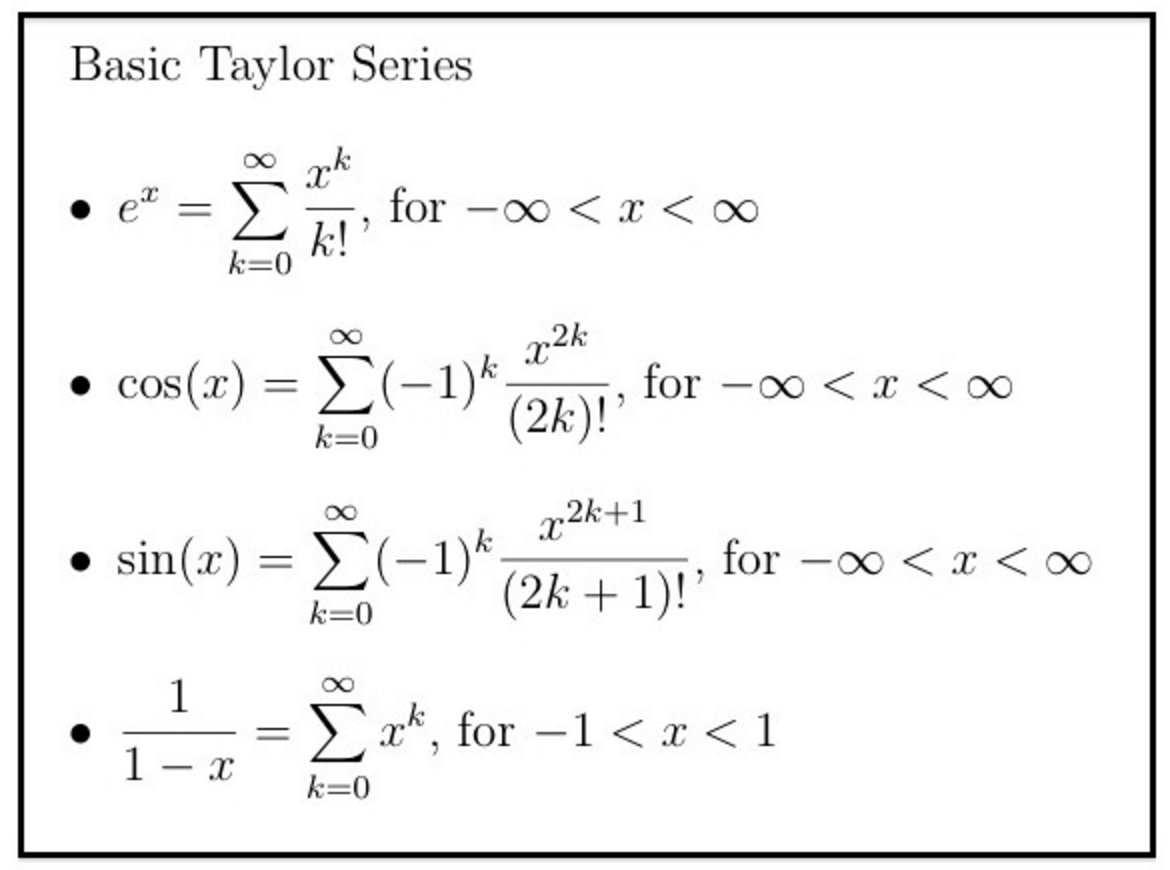
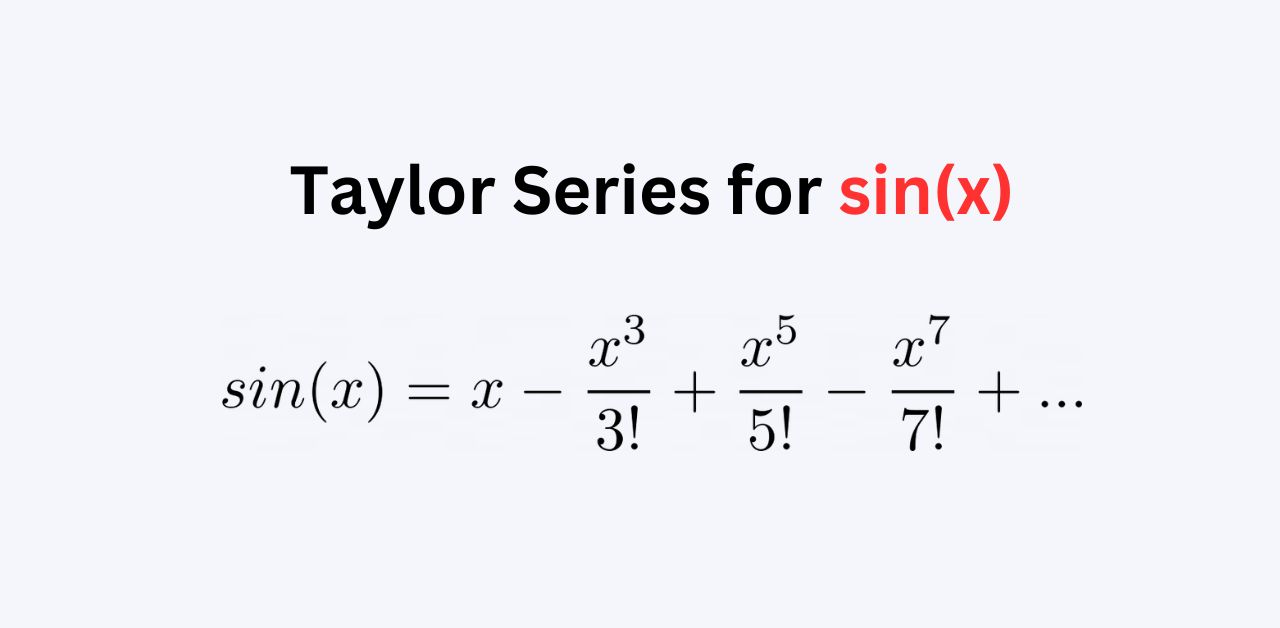Sinx Taylor Series

Sinx Taylor Series - See why this series is useful for. Learn how to expand a function into an infinite sum of terms using derivatives. See examples of taylor series for ex, sin, cos and other functions. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. I understand that taylor series expansion for $\sin(x)$ is derived as follow:

See examples of taylor series for ex, sin, cos and other functions. I understand that taylor series expansion for $\sin(x)$ is derived as follow: In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. Learn how to expand a function into an infinite sum of terms using derivatives. See why this series is useful for. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence.

Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. See examples of taylor series for ex, sin, cos and other functions. Learn how to expand a function into an infinite sum of terms using derivatives. I understand that taylor series expansion for $\sin(x)$ is derived as follow: See why this series is useful for. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the.

Find the Taylor’s series of sin⁡x in powers of (x π/2) up to the

Learn how to expand a function into an infinite sum of terms using derivatives. See why this series is useful for. See examples of taylor series for ex, sin, cos and other functions. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. I understand that taylor series.

Solved Use this list of Basic Taylor Series to find the

See examples of taylor series for ex, sin, cos and other functions. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. I understand that taylor series expansion for $\sin(x)$ is derived as follow: See why this series is useful for. Learn how to expand a function into an infinite.

Maclaurin Series for sin x (Calculus 2) YouTube

In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. See examples of taylor series for ex, sin, cos and other functions. I understand that taylor series expansion for $\sin(x)$ is derived as follow: Learn how to use taylor's formula to find the power series expansion of sin.

BC Taylor Series for sinx at 0.5pi YouTube

See why this series is useful for. Learn how to expand a function into an infinite sum of terms using derivatives. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. Learn how to use taylor's formula to find the power series expansion of sin x and its.

Derivation of the Taylor series expansion for the sine function YouTube

I understand that taylor series expansion for $\sin(x)$ is derived as follow: See examples of taylor series for ex, sin, cos and other functions. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. Learn how to use taylor's formula to find the power series expansion of sin.

163 11 10 Taylor Series sinx YouTube

See why this series is useful for. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. See examples of taylor series for ex, sin, cos and other functions. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to.

Taylor Series for sin(x) Howto & Steps Lesson

See why this series is useful for. In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. See examples of taylor series for ex, sin, cos and other functions. I understand that taylor series expansion for $\sin(x)$ is derived as follow: Learn how to use taylor's formula to.

Taylor Series for sinx

Learn how to expand a function into an infinite sum of terms using derivatives. See examples of taylor series for ex, sin, cos and other functions. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. In the next example, we find the maclaurin series for $e^x$ and $\sin x$.

Sin X Taylor Series slideshare

I understand that taylor series expansion for $\sin(x)$ is derived as follow: See why this series is useful for. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. Learn how to expand a function into an infinite sum of terms using derivatives. In the next example, we find the.

TAYLORS SERIES EXPANSION OF SINX AND COSX YouTube

I understand that taylor series expansion for $\sin(x)$ is derived as follow: See examples of taylor series for ex, sin, cos and other functions. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. Learn how to expand a function into an infinite sum of terms using derivatives. See why.

See Why This Series Is Useful For.

In the next example, we find the maclaurin series for $e^x$ and $\sin x$ and show that these series converge to the. Learn how to expand a function into an infinite sum of terms using derivatives. Learn how to use taylor's formula to find the power series expansion of sin x and its radius of convergence. I understand that taylor series expansion for $\sin(x)$ is derived as follow: