Strong Induction Discrete Math
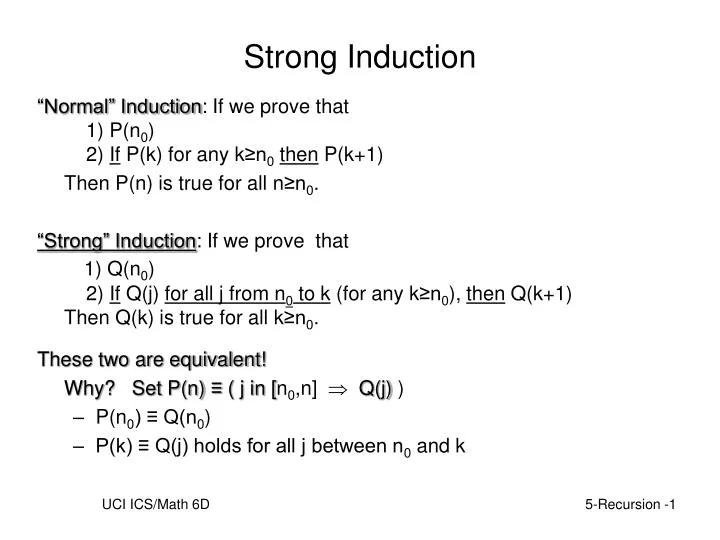
Strong Induction Discrete Math - Anything you can prove with strong induction can be proved with regular mathematical induction. We do this by proving two things: It tells us that fk + 1 is the sum of the. To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci numbers. Explain the difference between proof by induction and proof by strong induction. Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. We prove that p(n0) is true. Use strong induction to prove statements. Is strong induction really stronger? We prove that for any k n0, if p(k) is true (this is.
We prove that p(n0) is true. It tells us that fk + 1 is the sum of the. Anything you can prove with strong induction can be proved with regular mathematical induction. To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci numbers. Use strong induction to prove statements. We do this by proving two things: We prove that for any k n0, if p(k) is true (this is. Is strong induction really stronger? Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. Explain the difference between proof by induction and proof by strong induction.
We do this by proving two things: To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci numbers. We prove that p(n0) is true. Is strong induction really stronger? Explain the difference between proof by induction and proof by strong induction. We prove that for any k n0, if p(k) is true (this is. Anything you can prove with strong induction can be proved with regular mathematical induction. Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. It tells us that fk + 1 is the sum of the. Use strong induction to prove statements.
PPT Strong Induction PowerPoint Presentation, free download ID6596
To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci numbers. Anything you can prove with strong induction can be proved with regular mathematical induction. We prove that p(n0) is true. Is strong induction really stronger? We do this by proving two things:
PPT Mathematical Induction PowerPoint Presentation, free download
We prove that p(n0) is true. It tells us that fk + 1 is the sum of the. We prove that for any k n0, if p(k) is true (this is. Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. We.
SOLUTION Strong induction Studypool
Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. It tells us that fk + 1 is the sum of the. Is strong induction really stronger? We do this by proving two things: Use strong induction to prove statements.
induction Discrete Math
Is strong induction really stronger? Explain the difference between proof by induction and proof by strong induction. Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. To make use of the inductive hypothesis, we need to apply the recurrence relation of.
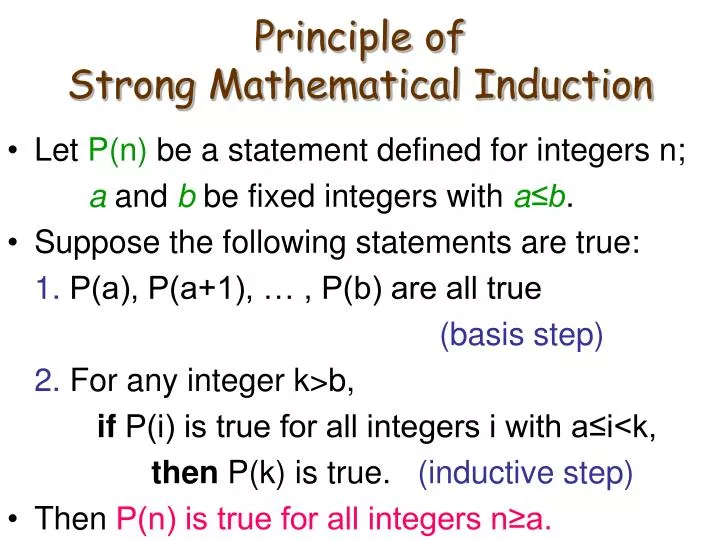
PPT Principle of Strong Mathematical Induction PowerPoint
Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. Is strong induction really stronger? Use strong induction to prove statements. Anything you can prove with strong induction can be proved with regular mathematical induction. We do this by proving two things:
Strong Induction Example Problem YouTube
Use strong induction to prove statements. It tells us that fk + 1 is the sum of the. Explain the difference between proof by induction and proof by strong induction. We do this by proving two things: We prove that p(n0) is true.
PPT Mathematical Induction PowerPoint Presentation, free download
Explain the difference between proof by induction and proof by strong induction. Is strong induction really stronger? We do this by proving two things: We prove that for any k n0, if p(k) is true (this is. Anything you can prove with strong induction can be proved with regular mathematical induction.
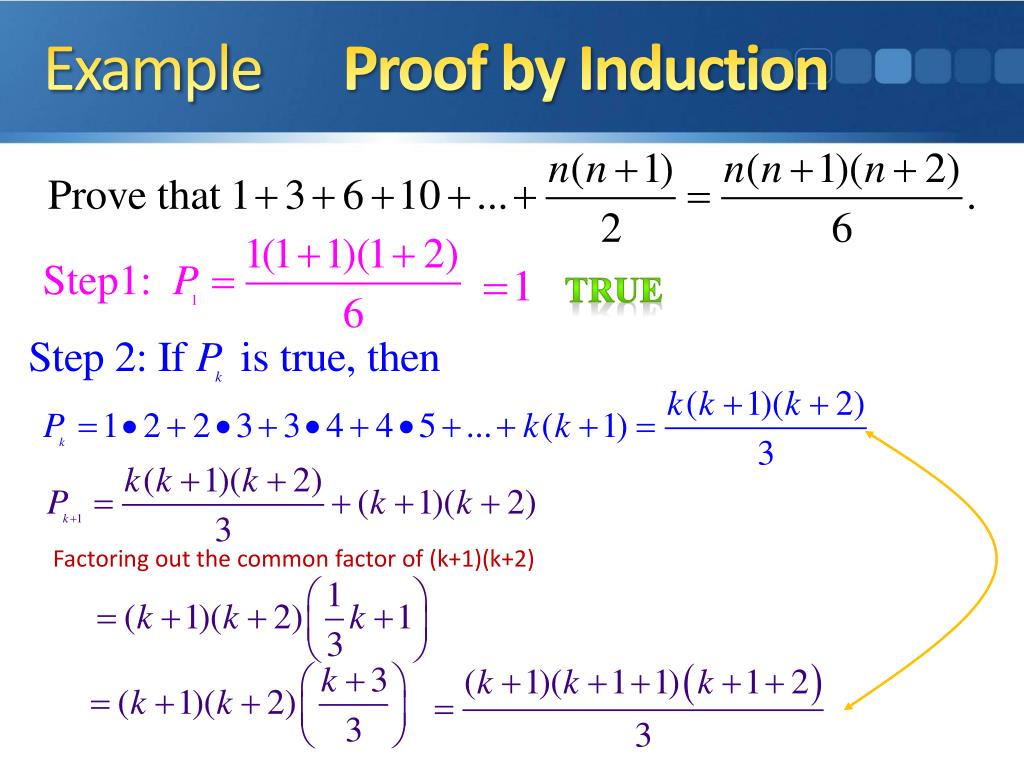
Strong induction example from discrete math book looks like ordinary
Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. We prove that p(n0) is true. To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci numbers. It tells us that fk + 1 is the.
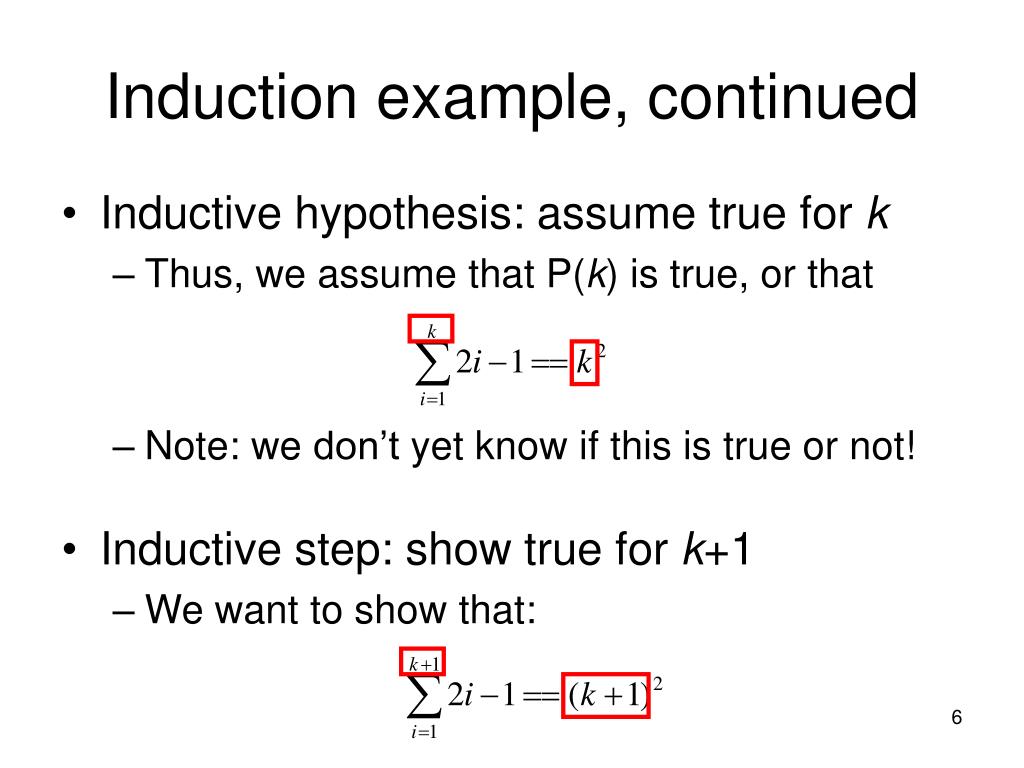
2.Example on Strong Induction Discrete Mathematics CSE,IT,GATE
Is strong induction really stronger? Explain the difference between proof by induction and proof by strong induction. We prove that for any k n0, if p(k) is true (this is. Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have. We prove.
PPT Mathematical Induction PowerPoint Presentation, free download
We prove that for any k n0, if p(k) is true (this is. Anything you can prove with strong induction can be proved with regular mathematical induction. Is strong induction really stronger? It tells us that fk + 1 is the sum of the. To make use of the inductive hypothesis, we need to apply the recurrence relation of fibonacci.
We Prove That P(N0) Is True.
It tells us that fk + 1 is the sum of the. We prove that for any k n0, if p(k) is true (this is. We do this by proving two things: Explain the difference between proof by induction and proof by strong induction.
To Make Use Of The Inductive Hypothesis, We Need To Apply The Recurrence Relation Of Fibonacci Numbers.
Use strong induction to prove statements. Anything you can prove with strong induction can be proved with regular mathematical induction. Is strong induction really stronger? Now that you understand the basics of how to prove that a proposition is true, it is time to equip you with the most powerful methods we have.