What Is The Reference Angle For 5Pi 3
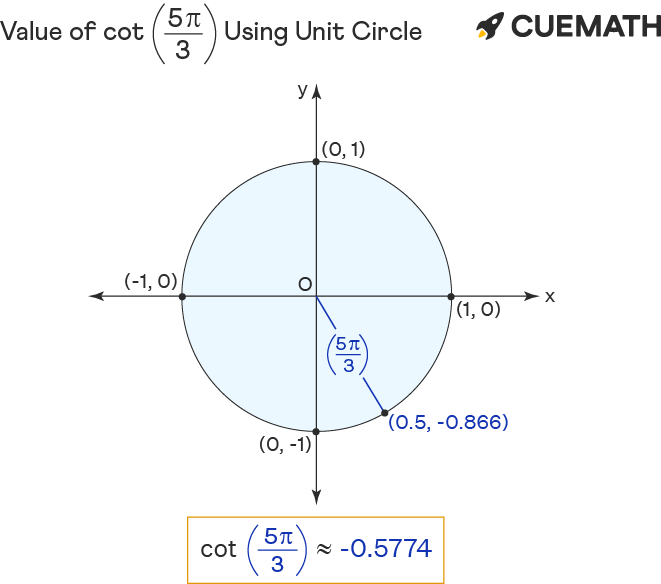
What Is The Reference Angle For 5Pi 3 - Given, angle in radians = 5π/3. The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference angle is 60 degrees with respect to the horizontal. The angle is, ⇒ 5π / 3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. Therefore the correct option is b. The reference angle for 5π/3 is π/3. Here, we can clearly see that. The reference angle of 3π/4 will be π/3.
The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference angle is 60 degrees with respect to the horizontal. The reference angle for 5π/3 is π/3. Given, angle in radians = 5π/3. Here, we can clearly see that. The angle is, ⇒ 5π / 3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. The reference angle of 3π/4 will be π/3. Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. Therefore the correct option is b.
The reference angle of 3π/4 will be π/3. Given, angle in radians = 5π/3. Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. Therefore the correct option is b. The angle is, ⇒ 5π / 3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. The reference angle for 5π/3 is π/3. The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference angle is 60 degrees with respect to the horizontal. Here, we can clearly see that.
Cot 5pi/3 Find Value of Cot 5pi/3 Cot 5π/3
Here, we can clearly see that. The angle is, ⇒ 5π / 3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. The reference angle for 5π/3 is π/3. The reference angle of 3π/4 will be π/3.
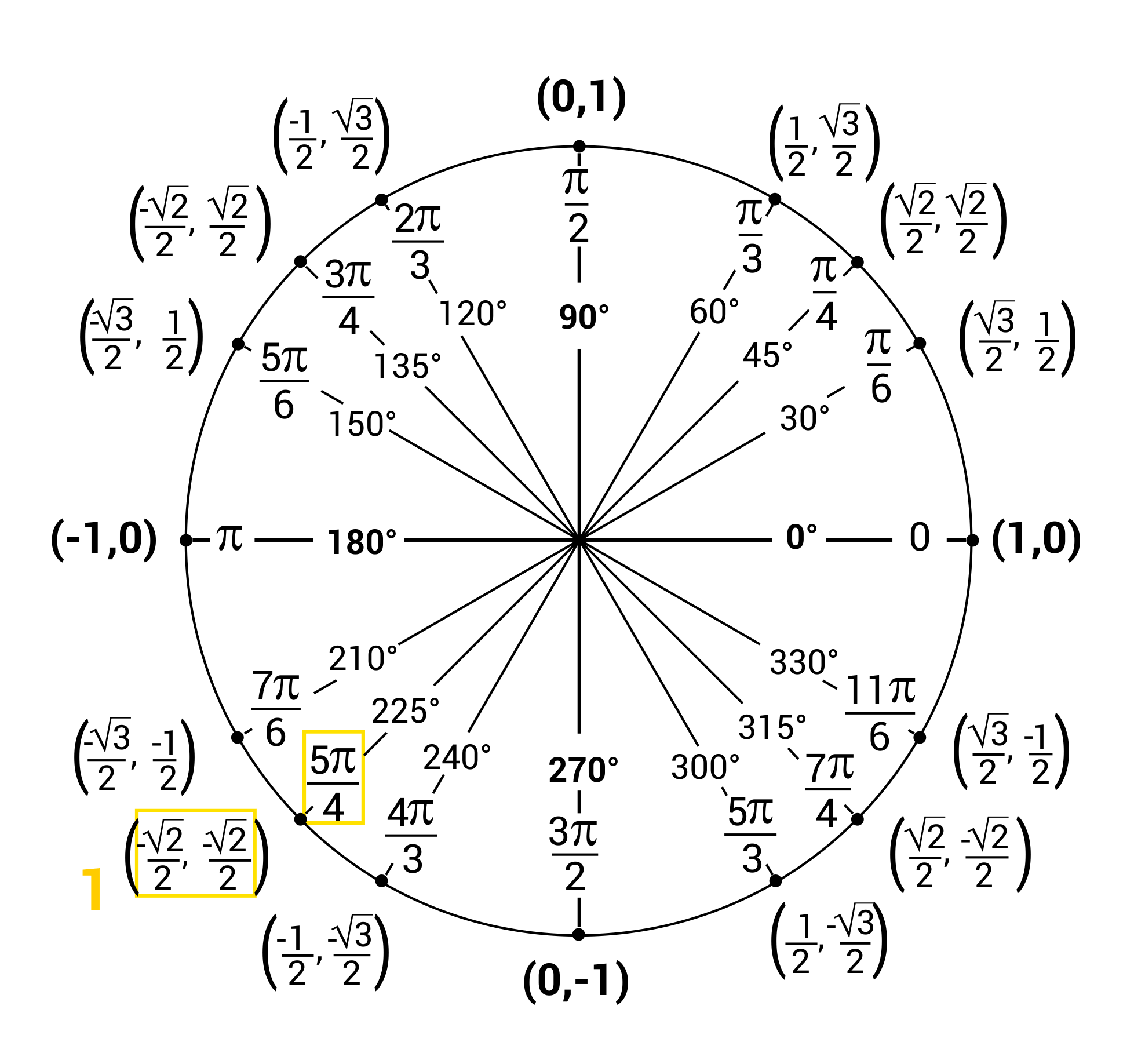
Unit Circle Practice Worksheets
Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. Given, angle in radians = 5π/3. The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and.
The reference angle for (5pi)/4 is pi/4 , which has a terminal point of
The reference angle of 3π/4 will be π/3. The angle is, ⇒ 5π / 3. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. The reference angle for 5π/3 is π/3. The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference.
for this special angle, draw the angle and find the reference angle t
Here, we can clearly see that. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. The reference angle for 5π/3 is π/3. The reference angle of 3π/4 will be π/3. Given, angle in radians = 5π/3.
Ex Sine And Cosine Values Using The Unit Circle Multiples, 54 OFF
The reference angle of 3π/4 will be π/3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference angle is 60 degrees.
Angle Of Rotation Examples
Therefore the correct option is b. The reference angle of 3π/4 will be π/3. The angle is, ⇒ 5π / 3. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. The reference angle for 5π/3 is π/3.
inverse trigonometric functions and reference angles for the following
The reference angle for 5π/3 is π/3. Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. The angle is, ⇒ 5π / 3. The reference angle of 3π/4 will be π/3. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full.
pi/3 is the reference angle for A. 2pi/3 B. 15pi/3 C. 7pi/3 D. 19pi/3
The reference angle of 3π/4 will be π/3. The reference angle for 5π/3 is π/3. Therefore the correct option is b. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full. Here, we can clearly see that.
What is the reference angle and cosine of StartFraction 7 pi Over 6
Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. Therefore the correct option is b. The reference angle for 5π/3 is π/3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. Given, angle.
Reference Angles NBKomputer
Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. The reference angle of 3π/4 will be π/3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle. The angle 5pi/3 is in the fourth.
Here, We Can Clearly See That.
The reference angle of 3π/4 will be π/3. Since 5π/3 radians is more than π (180°), it is located in the fourth quadrant of the unit. Therefore the correct option is b. To find the reference angle for 5π/3, we need to determine the equivalent angle within one full.
Given, Angle In Radians = 5Π/3.
The angle 5pi/3 is in the fourth quadrant (meaning cosine is positive while sine & tangent are negative), and its reference angle is 60 degrees with respect to the horizontal. The reference angle for 5π/3 is π/3. The angle is, ⇒ 5π / 3. The latter is known as the vertex of the angle and the rays as the sides, sometimes as the legs and sometimes the arms of the angle.